Spheres vs. aspheres
Differences & application areas
内容
Geometry, advantages and fields of application of spherical and aspheric optics
One of the lens shapes most frequently requested on the optics market is still the sphere. Due to its proven design and precise manufacturing possibilities, it meets high optical quality standards. In the meantime, the economical and high-precision production of aspheres is also feasible. The use of aspheric lenses in modern systems, such as medical technology or laser material processing, has only become possible thanks to state-of-the-art technology. Until today, the production of both types of lenses could be further optimized by sophisticated CNC technology, intelligent control software and interferometric measuring techniques. Differences between spheres and aspheres are mainly found in their geometries and the resulting complexity of their production and measurement.
Spherical lenses
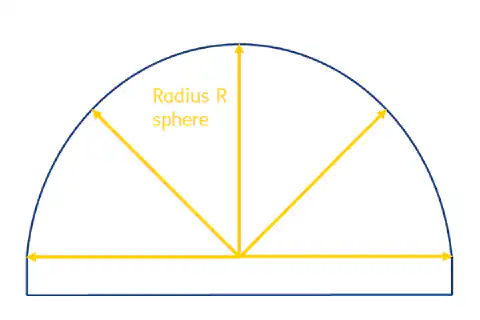
Spheres are rotationally symmetric optics whose shape corresponds to the section of a spherical surface (Fig. 1). The radius of curvature has an unchanged distance from the geometrical center. This means the optically effective surface can be described by specifying only one parameter, the radius R. Since this parameter is constant over the entire surface, spheres offer cost advantages in manufacturing.
Figure 1: Description of the optically effective area of a sphere by means of radius
Manufacturing advantages of spheres
In terms of production costs, the sphere achieves clear advantages. This is due to its geometry. The uniform shape of the sphere surface ensures an uncomplicated manufacturing process and shorter production times, especially for small diameters, since several optics are manufactured simultaneously on one supporting body. The same applies to the processes of optical inspection and measurement, since uniform and thus quickly generated results can be measured over the entire surface. Tactile measuring methods (e.g. profilometer or 3D coordinate measuring machine), but also optical measuring methods such as interferometers and computer-generated holograms (CGH) are used for the measurement of spherical surfaces. As with other optics, the choice of measuring instrument is based on a comparison of cost and benefit to be able to decide which method to use.
Application areas for spheres
Spheres are used in a wide range of applications, e.g. in metrology, aerospace (spectrometers installed inside satellites) or medical technology (slit lamps for the examination of the anterior segment of the eye). Due to the combination of low manufacturing costs, fast production times and a wide range of optical applications, spheres are an integral part of the optical market and convince with a very good price-performance ratio.
Application optimization of spherical single lenses
Depending on the shape, collecting, scattering or focusing properties of the sphere are used to refract incident light to the desired degree. In imaging systems, for example, high image quality plays a decisive role and is accompanied by low imaging errors. In addition, it can be increased - depending on the requirements of the existing system - by taking various factors into account. These include, for example, the position of the light source used or the choice of an effective aperture. The image quality can also be improved by using several spheres, but this is a question of the lens shape and the existing space conditions of the optical system. By choosing an effective aperture, it is also possible to reduce the spherical aberration. Reason for this is the blocking of the peripherally incident rays. Without an aperture, the peripherally increasing curvature and the resulting stronger refraction of the rays promotes the development of spherical aberrations.
Combination of several spherical lenses
An achromat is created by combining one or more collecting and dispersing lenses. Usually a positive convex lens of low refractive index (crown glass) and a negative concave lens of low refractive index (flint glass) are used and cemented together. This results in an optical system that improves the spherical aberration as well as the chromatic aberration. Achromats are used, for example, in the field of photography, within photographic lenses.
Aspheric lenses
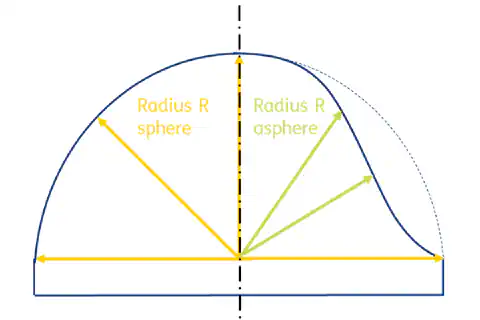
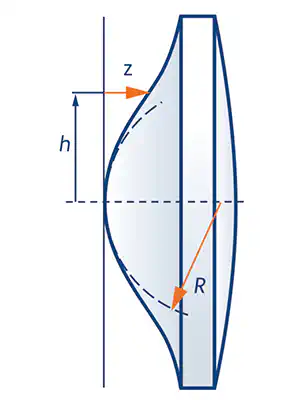
If various factors, such as high image quality, numerical aperture or maximum space saving must be considered within an optical setup, the asphere is the best choice. Aspheric lenses are rotationally symmetrical optics whose radius of curvature deviates radially from the center of the lens. Thanks to this special surface geometry, aspheres can significantly increase the imaging quality of optical systems compared to spherical lenses. Their different radii of curvature cause the deviation from a spherical surface (Fig. 2).
Figure 2: Description of the optically effective area of a sphere compared to an asphere
The deviation from the spherical shape becomes apparent on closer inspection of the flattening radii towards the lens periphery. In general, the following statement applies: A lens is an asphere as soon as its radius deviates from the spherical shape. The radius of the lens is determined in such a way that - as can be seen in Fig. 3 - there is a bundling of the incident rays which intersect at a common focal point and thus prevent spherical aberration. An asphere is therefore an optimized focusing optic. In comparison, the sphere whose incident rays are deflected much more strongly with increasing distance from the optical axis and do not meet at one common point (Fig. 3). The result of aberrations caused by a sphere is slightly blurred, unsharp images. Aspheres can therefore be used to improve the image quality.
Figure 3: Correction of spherical aberration by an asphere
Mathematical description of aspheric surfaces
Regarding their optical design, aspheric surfaces have a higher number of degrees of freedom compared to spheres, which means that more complex surfaces can be created. The optically effective surface of rotationally symmetric aspheres is traditionally defined by the following asphere formula:
With the following breakdown of the parameters:
z = Sag of surface
h = Distance perpendicular to the optical axis (height of incidence)
R = Radius
k = Conic constant
A2i = Aspheric coefficients of the correction polynomial
If the asphere coefficients are zero, the surface shape corresponds to a rotationally symmetrical conical section. This is expressed as follows:
| Conical constant | Conic section |
|---|---|
| k = 0 | Sphere |
| k > -1 | Ellipsoid |
| k = -1 | Parabola |
| k < -1 | Hyperbola |
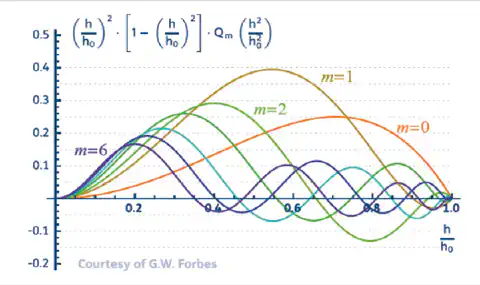
Since the renewal of ISO 10110, published in 2015, there is an alternative description for aspheric surfaces. It is based on a set of orthonormal polynomials, so-called Qbfs polynomials, which model the deflection difference to the best-fitted sphere of the asphere. The surface quotient is given in the following formula:
The advantage of the new description is that less significant digits are required to describe the surface shape. In addition, the maximum deflection deviation can be estimated by multiplying the largest coefficient Am by the maximum amplitude for the order of this coefficient (see Fig. 4).
Figure 4: Graphical description of Qm
Reduction of an optical system by aspheres
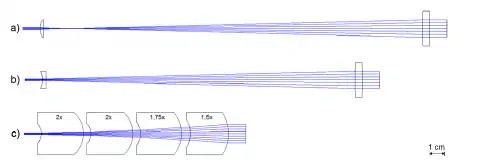
A further advantage of aspheric lenses compared to conventional lenses is the reduction in the overall length of an optical system. An example can be found in the field of beam expansion, with the monolithic beam expander from asphericon. Consisting of only one single lens, an afocal system could be realized by aspherization of one of the two lens surfaces, which can expand beams, even larger beam diameters, without opening errors. Due to the afocal character of this system, several monoliths can be connected in a row. This allows a reduction of the optical system while, at the same time, varying the total beam diameter. The beam expansion system a|BeamExpander developed by asphericon shortens the total length by up to 50% compared to conventional systems. The following figure shows a Kepler and Galilean telescope with 10x magnification (M=10). This is compared to the a|BeamExpander with identical magnification but halved in length.
Figure 5: a|BeamExpander compared to Kepler and Galilean telescopes
The phenomenon of system reduction can also be found in other optical arrangements, e.g. within photographic lenses. An additional advantageous side effect is the reduction in weight. In the case of “every gram counts”, enormous savings can be achieved, for example in inspection by satellites such as the Sentinel-4 satellite. Initiated by the Copernicus program of EU and ESA, the Sentinel-4 satellite provides reliable real-time data for the environmental management of Europe and North Africa through two high-resolution spectrometers.
Production and measurement of aspheres
Just like spheres, aspheres can also be produced by various methods, e.g. by grinding and polishing. For a long time, it was a myth that aspheric lenses were only suitable for laboratory, R&D projects, or prototype construction and that their use in larger quantities was not economical. With modern manufacturing and measuring technologies, aspheres can also be produced in series with reproducible accuracy. By increasing the batch size, the set-up costs are distributed, which ultimately leads to lower unit prices.
The completely digitalized production world at asphericon is unique worldwide. All process, information, and manufacturing steps from the first customer contact to the shipment of the final optical system are digitally controlled by a software-based control tool developed in-house (Fig. 6). The production flow can thus be significantly optimized, yield be increased by simple data analysis (target/actual) and data transport be organized without losses. This is accompanied by the increasing automation of manufacturing processes and the digital control of supplier and logistics processes.
Figure 6: Schematic manufacturing process of asphericon based on its own control software
Due to a high degree of flexibility regarding the selection of tools, the range of feasible optical shapes has also increased significantly. Thus, the geometry of an aspheric lens has a decreasing effect on costs. In addition to the choice of material and the diameter of an optic, surface form deviation and quality of the surface are the main factors that affect manufacturing costs.
The measurement of aspheric lenses has also become much faster and less complicated in recent years. Technologies such as measurement with a CGH, interferometric methods and tactile measurement using probes have been further optimized, as has the manufacturing process itself. In addition, new measuring methods have been developed, such as tilted wave interferometry. This process uses differently tilted wavefronts and enables the measurement of optical surfaces in only 20 to 30 seconds. The measuring system acquires optics in many subapertures without contact, combines the interference patterns of these into a surface topography and determines the deviations from the target shape.
Use of aspheres
Thanks to their ability to correct spherical aberrations, among other things, aspheres can be found in a wide range of applications, e.g. in metrology and imaging, as well as within laser applications (see section “Reduction of an optical system by aspheres” with an example of laser beam expansion). They complement, for example, modern fluorescence microscopes, projection systems or the optical setup of a laser system. Due to the special advantage of system reduction, given by the replacement of spherical lenses by aspheres in optical systems, the weight can be reduced additionally, which plays a decisive role e.g. in the field of aerospace. By reducing weight, for example, fuel consumption can be lowered when sending an earth observation satellite.
Spheres vs. aspheres - A final comparison
Aspheres clearly dominate in terms of imaging quality, but this is still reflected in the higher production/measurement effort and thus higher costs compared to the spherical lens. However, this is counteracted by the saving of individual lenses. The following table shows a comparison of both lens geometries.
| Spheres | Aspheres | |
|---|---|---|
| Symmetry | Rotationally symmetric | Rotationally symmetric |
| Distance of the radius of curvature from the center | Equal | Extremely variable |
| Surface shape |  Bi-convex - Convex/plan - Convex-concave (converging meniscus) - Convex-concave (diverging meniscus) - Plan-concave - Bi-concave | |
| Spherical aberration correction | Restrictedly possible | Possible |
| Comparison of production and control expenditure | Less | Higher |
| Metrology | Optically and tactile, 2D and 3D | Optically and tactile, 2D and 3D |
| Cost comparison | Lower | Higher |
The combination of both lens types can also achieve very good imaging properties, which is particularly convincing in terms of the price-performance ratio, as well as the size and weight of the optical setup. Since spheres are usually the cheaper alternative, it is often tried to keep the number of aspheres as low as possible and still achieve very good imaging results. Important for the decision which lens type is best suited for an optical task depends on the respective application and the optical requirements on the imaging quality. From a manufacturing point of view, it can be predicted that - as a result of constantly improved software and the progressive development of manufacturing technology in the production sector - factors such as the processing time between spheres and aspheres will increasingly converge. The focus will shift more and more to surface quality. This will have a particularly positive effect on customer-specific requirements since numerous optical tasks can be solved more and more effectively as surface quality increases. The requirements of new technologies based on high-quality optics will continue to increase. Fields of application such as aerospace or exploration of the seabed for economic cultivation are coming more and more to the fore and require ever more sophisticated and powerful optics. Spherical and aspheric optical systems will continue to play a decisive role in the economically efficient realization of new applications based on photonic solutions.